Games of conflict and coordination – The tug-of-war of economic life

The game of life

The game of economic and biological life is one of continual conflict and coordination. A symbiotic web of interdependencies alongside a perpetual struggle for survival and progress. Humankind, in particular, displays incredible capacity for cooperation and competition at many levels of social organization.

From bridges to airplanes, people pool their knowledge and specialities to produce all sorts of sophistication. Others, meanwhile, will set up next door to compete for a slice of that economic pie. Nations too have shown impressive feats of collaboration, from international trade to scientific advancement. And by equal measure, as much potential for mutually assured destruction as well.

Humans are at constant odds with themselves, a tug-of-war between interest in the self and the collective. Many games of strategic interdependence – like the Game of Chicken, Stag-Hunt, and Prisoner’s Dilemma – contain elements of mutual interest and conflict. An understanding of these tensions can provide insights into human behavior and strategic thinking. In this post, we introduce two more games on opposite extremes: (1) A game of pure conflict; and (2) a game of coordination.

Jump ahead

Pure conflict (zero or constant-sum games)
A game of coordination

Pure conflict (zero or constant-sum games)

In games of pure conflict, a strategy profile that makes one player better off tends makes another worse off. Economists sometimes refer to these games as constant-sum or zero-sum games, where the sum payoff total under each strategic profile is always the same (or zero in the case of zero-sum games).

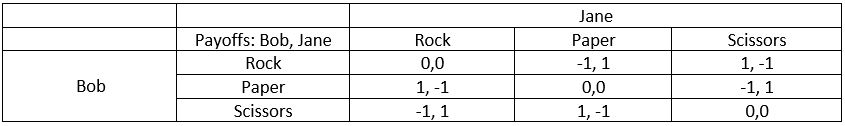
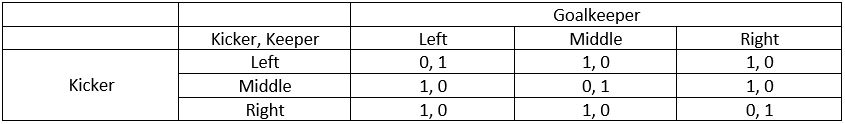
Rocks and penalties

Rock-paper-scissors and penalty kicks (in football) are simple examples of pure conflict. When one player wins, the other loses. And neither player has a dominant nor dominated strategy. We cannot find a strategic profile in which every player is using their best response given what the other is doing. As such, no Nash equilibrium in pure strategies exists. Whatever strategic profile they play, someone has an incentive to change what they’re doing. Games of pure conflict involves outguessing or outmaneuvering your opponent. (While games of pure conflict have no solution in pure strategies, there is another solution concept to draw upon: Nash equilibrium in mixed strategies. Indeed, many players arrive at this solution instinctively through randomized play – which we discuss in more detail in a later post.)

Game of pure conflict (rock-paper-scissors)

Game of pure conflict (penalty kicks)

A game of coordination

As its name implies, coordination games are strategic situations in which players stand to benefit from cooperation. It is a game of mutual interest. If players could communicate and coordinate beforehand, we’d expect players to arrive at a stable, agreeable solution.

Food and drinks

In the coordination game below, Vincent and Elinor have to decide what they bring to a picnic. Unfortunately, both players are too shy to contact each other prior to the picnic. Of course, both Vincent and Elinor would like some mix of food and drinks. And neither want too much food or too many drinks.

Coordination game (picnics)

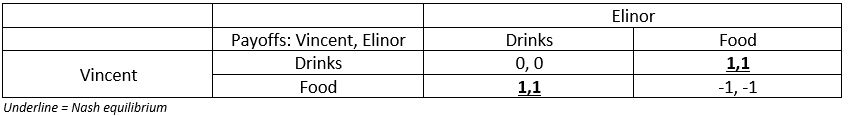
In this symmetric game, there are two Nash equilibria: (Drinks, Food) and (Food, Drinks). If the strategic profile contains a mix of food and drinks, neither player has an incentive to change their strategy. Hence, a Nash equilibrium. By contrast, the strategic profile that contains a doubling of food or drinks is unstable, both players have an incentive to change their strategy.

We can find games of coordination everywhere. When you are guessing as to how formal to dress for some party or social event, you’re in a game of coordination. The Stag-Hunt game, which we discussed previously, is another a game of coordination. Likewise, the Prisoner’s Dilemma contain elements of cooperation. Both players would probably cooperate if there was a credible, cost-effective mechanism to foster coordination.