Nash equilibrium – A fundamental solution concept and hallmark of game theory

AuthorAthenarium / Posted on

Nash equilibrium – A fundamental solution concept, and the hallmark of game theory

Nash and stags

Previously, we discussed how searching for dominant strategies, if they exist, can help us to simplify and solve some games. This post introduces another solution concept: the Nash equilibrium – the cornerstone of game theory, named after the brilliant John Nash Jr. This post will state the intuition behind the Nash equilibrium first, unpack its assumptions second, and use the classic Stag-Hunt game to tie some ideas together.

Skip ahead

Nash equilibrium

A strategy profile – a profile that specifies the strategy that each player is using – is a Nash equilibrium if every player is using their best response given the strategy that everybody else is playing. That is, at this strategy profile, no player has an incentive to change his or her strategy given what everybody else is doing.

“A Nash equilibrium is a configuration of strategies where each player’s choice is his best response to the other player’s choice (or the other players’ choices when there are more than two players in the game). If some outcome is not a Nash equilibrium, at least one player must be choosing an action that is not his best response. Such a player has a clear incentive to deviate from that action, which would destroy the proposed solution.”

Avinash Dixit, & Barry Nalebuff. (2008). The Art of Strategy.

Best responses

The best response is a strategy that maximizes the player’s individual payoff, given the beliefs they have about the strategies that other players will use. It follows that to find a Nash equilibrium, we must look for the best responses of each player. If a dominant strategy exists, that strategy is the player’s best response. Conversely, if a dominated strategy exists, then that strategy is never the player’s best response. And if there are no dominant or dominated strategies, then the player’s best response may vary with the action of others.

“The idea is to look for an outcome where each player in the game chooses the strategy that best serves his or her own interest, in response to the other’s strategy. If such a configuration of strategies arises, neither player has any reason to change his choice unilaterally. Therefore, this is a potentially stable outcome of a game where the players make individual and simultaneous choices of strategies.”

Avinash Dixit, & Barry Nalebuff. (2008). The Art of Strategy.

Stag Hunt game

Here, we introduce the classic Stag Hunt game. In its simplest form, two hunters, Yosemite and Elmer, must simultaneous and independently decide whether to hunt for hares or stags. Hare hunting is a lonesome venture that guarantees a small payoff. Stag hunting, by contrast, requires coordination. Both hunters must partake together to succeed. The strategies and options of both hunters are summarised below. What are the solutions to this game?

Stag Hunt game

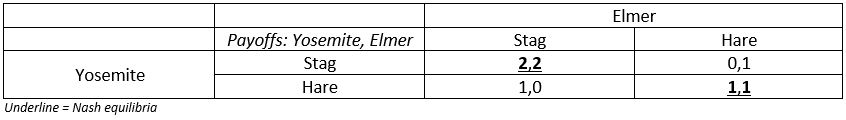
Nash equilibria in pure strategies

There are two Nash equilibria in pure strategies in this symmetric game.^ The first Nash equilibrium has both hunters seeking hares. If Elmer believes that Yosemite is hunting hare, then it’s in Elmer’s best interest to hunt hare as well (and vice versa). The second Nash equilibrium has both players hunting stags. If Yosemite believes that Elmer is hunting for stag, then it’s in his best interest to hunt stag too (and vice versa once again).

Both these strategic profiles are Nash equilibrium because neither hunter has an incentive to change their strategy given what the other is doing. If both players are pursuing stag, neither wants to switch to hares. Likewise, if both hunters are pursuing hares, neither wants to switch to stags for a zero payoff. Note also that the other strategic profiles in which Elmer and Yosemite choose different animals are not Nash equilibrium. In these situations, both hunters can earn a higher payoff by switching their strategy to the animal that they believe the other is hunting.

^ It is pure strategies in the sense that each player has a unique response to the actions of others. In more advanced topics, players might introduce mixed strategies that randomise responses to a given action. We refer to the solution concept associated with mixed strategies as a Nash equilibrium in mixed strategies. In this post, we focus only on Nash equilibria in pure strategies.

Beliefs and coordination

Best responses may give the impression that players are deciding sequentially. Do remember, though, that this is actually a simultaneous game. Players are simply formulating beliefs about what to do, given what they believe the other might do. The Stag Hunt game, in particular, is a game of coordination. Each hunter wants to do what he believes the other is doing. While the equilibrium in which both players pursue the stag is superior, we cannot say which equilibrium might emerge without further information.

Rationality and accurate beliefs

The Nash equilibrium depends on two assumptions. Firstly, it assumes that all players are rational. Players take actions to maximize their payoffs given their beliefs about the behaviors of everyone else. Secondly and relatedly, it assumes that the beliefs players hold about other players are correct. In our example above, both players are rational, and believed the other rational too. If the players formulated beliefs and response rules to payoffs differently, we might get a different solution or no solution at all.

Rest points and focal points

A Nash equilibrium is a stable solution to the game because every player is maximizing their payoffs, based on the correct beliefs they hold about others. If a Nash equilibrium exists, and every player is using their Nash equilibrium strategy, then nobody has any incentive to do things differently. Some textbooks refer to this solution as a ‘rest point’ of the game.

Multiplicity

As the Stag Hunt game illustrates, some games may have two or more Nash equilibria. And it’s difficult to say what will happen when multiple solutions exist. Perhaps, if the game is repeated, players might develop recurring habits, rules and beliefs. But this doesn’t tell us much about the solution in the very first game.

In The Strategy of Conflict, game theorist Thomas Schelling suggests that focal points might exist in the context of the game. This is where history, experiences, culture, language, personalities, whims, fads, and so on, generate tendencies in beliefs and expectations. This, in turn, may bias one equilibrium over another, especially if this focal point is common knowledge.

“Many mathematical game theorists dislike the dependence of an outcome on historical, cultural, or linguistic aspects of the game … they would prefer the solution be determined purely by the abstract mathematical facts about the game … We disagree. We think it entirely appropriate that the outcome of a game … depend on the social and psychological aspects of the game.”

Avinash Dixit, & Barry Nalebuff. (2008). The Art of Strategy.

Payoff dominance

You’ll notice also that the Nash equilibrium concept is based on individual rationality. That is, players pursue actions that maximize their payoffs based on what other players are believed to do. Other solution concepts might focus on collective rationality or some other selection mechanism. One example is payoff dominance. A Nash equilibrium payoff dominates another when it yields a strictly higher payoff for all players in the game. This is another potential solution mechanism, especially when multiple Nash equilibria exist.

Deep thinking

The Nash equilibrium and iterative deletion of strictly dominated strategies (IDSDS) are two solution concepts. While every Nash equilibrium will survive the IDSDS algorithm, not every strategy profile that survives IDSDS is necessarily a Nash equilibrium. In this way, the Nash equilibrium is a stricter solution concept.

But unlike the IDSDS method, there are no standard procedures for finding every Nash equilibrium. While shortcuts exist, they depend on the game-specific properties like symmetry. Thankfully, games in introductory courses aren’t too complex. So, full-proof methods like exhaustive searches tend to suffice.

In games like chess, however, exhaustive search isn’t feasible. This is so, even if you’re a chess grand master or supercomputer. There are just too many permutations to consider. To make decisions, chess grand masters have to rely not only on impressive calculations and memorized playbooks, but on general principles for move selection as well.

The point here is that the literature on decision-making goes well beyond the solution concepts discussed in elementary game theory. General principles and behavioral psychology, for example, can play important roles. For the interested, I highly recommend former chess grand master Garry Kasparov’s Deep Thinking as a starting point.

Further reading

Return to game theory home