The Prisoner’s Dilemma – An economic, political and evolutionary bastille

The Prisoner’s Dilemma is prevalent in many economic, social and political situations. From prison snitches to nuclear arms races, this post looks at a cornerstone problem of game theory.

Prisoner’s Dilemma

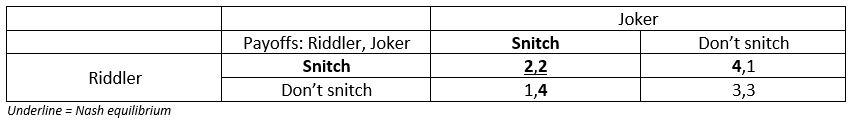
The Prisoner’s Dilemma, in its classic formulation, involves two criminals – let’s say the Riddler and Joker – that have just committed a crime. During separate interrogations with police, the Riddler and Joker must decide whether to snitch on one another for a lighter sentencing.

The dilemma has three outcomes. Firstly, if one snitches and the other does not, then the snitch receives the lightest sentence, while the other bares the heaviest punishment. Secondly, if both criminals snitch, then both of them share equally in a heavy punishment. Finally, if neither criminal snitch, then both get to walk away with a lighter sentence. We summarise the players, choices, and associated payoffs in the table as follows:

Prisoner’s Dilemma (game of snitching)

Nash equilibrium

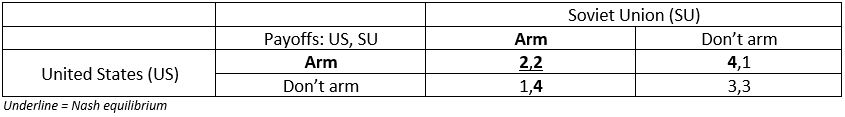
What’s the solution to this game? Let’s look at their best responses first. If the Riddler is snitching, then it is in the Joker’s best interest to snitch. And if the Riddler is not snitching, the Joker still wants to snitch to get a lighter punishment. Because the payoffs in this game are symmetric, the same arguments apply in reverse too. That is to say, both the Joker and the Riddler have a strictly dominant strategy to snitch on the other. Indeed, the unique Nash equilibrium to the Prisoner’s Dilemma sees both players snitching.

Games of expansion

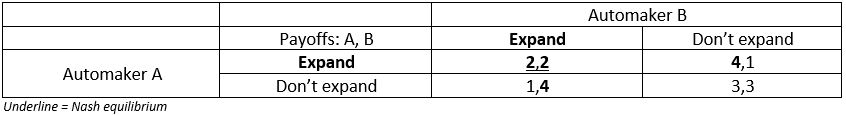
The Prisoner’s Dilemma occurs in many strategic settings. In business, companies often find themselves in profit-destroying price wars and expansionary projects. In the table below, there is a duopoly of automakers that prizes market share over all else – they find it intolerable if the other is expanding, and they are not. Because of their strictly dominant strategy to expand, relative market shares remain unchanged. In the end, both companies have committed more resources to little relative avail.

Prisoner’s dilemma (game of expansion)

Games of marketing

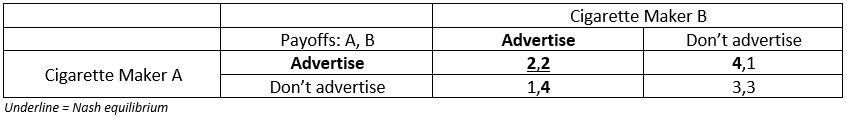
Similarly, consider a game of advertising between two cigarette brands in the table below. Each cigarette maker has an incentive to market themselves to attract new customers. This is most valuable if the other maker is not advertising. But if everybody is advertising, the end result, much like the expansion game above, is a Prisoner’s Dilemma in which resources are dedicated to little relative gain.

Prisoner’s dilemma (game of advertising)

Unfortunately, if every cigarette maker is advertising, we might need regulation to protect uninformed consumers. But a ban on cigarette advertising, as Scott Stevens points out in Games People Play, might help cigarette makers to escape their Prisoner’s Dilemma inadvertently!

Arms races

Prisoner’s Dilemmas are not uncommon to politics and diplomacy, either. Some politicians, for example, cannot help but attack their opponents during political campaigns. Restraint may not be a good strategy when your opponent has you on full blast. More worrisome is the problem of arms races, in which superpowers compete for military superiority. Yes, nuclear arms development is expensive. Taxpayers would rather dedicate national resources to education, healthcare and other domestic priorities. But what happens when your adversaries arm themselves, and you don’t? When nations believe they have a strictly dominant strategy to arm themselves, an arms race seems inescapable. If only we could channel that same motivational structure towards education outcomes, poverty alleviation and a climate change resolution, right?

Prisoner’s dilemma (arms race)

“Beliefs matter … If everybody believes, and expects everybody else to believe, that things get more dangerous when the first nuclear weapon goes off, … and in the event nuclear weapons are used, … [then everybody] will be expectant about rapid escalation in a way that could make escalation more likely. Virtually all of these thresholds are fundamentally matters of beliefs and expectations.”
Thomas Schelling. (1966). Arms and Influence.

Forest games

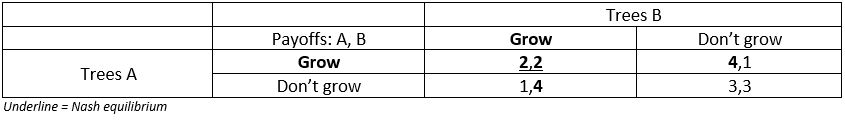
Prisoner’s Dilemmas happen in nature too. Some species of trees, for example, might find themselves in an arms race for height. After all, natural selection favours taller trees that enjoy more sunlight. As a result, surviving trees, and their progeny, may allocate more and more energy to vertical growth. Unfortunately, when every tree uses the same strategy, their relative performance remains largely unchanged. The result is a collective net loss since every tree is allocating more resources to height.

Prisoner’s dilemma (game of height)

Fatalism and extensions

The Prisoner’s Dilemma may appear fatalistic. Remember, however, that the Prisoner’s Dilemma is not the only payoff structure in games. If an arms race appears untenable, both sides have an incentive to transform the game. Game theory is as much about choosing the game as it is about playing it. Additionally, the examples above are simple, single shot variants of the Prisoner’s Dilemma. It is not the final word on the topic. The repeated Prisoner’s Dilemma, for example, can generate sustained cooperation despite the temptation to snitch, defect, or arm in a single shot game (under the right conditions of course). Conversely, the Prisoner’s Dilemma can manifest itself in larger collective-action problems like the famous Tragedy of the Commons.